The fact that isotopes have a unique half-life has proven perhaps humanity’s most powerful tool for understanding the deep history of our planet. But what is a half-life, how do we use them – and for that matter, what even is an isotope?
The nucleus of an atom is made up of protons and (aside from most hydrogen) neutrons. The number of protons defines what element it is: 8 for oxygen, 26 for iron, and 79 for gold. However, the number of neutrons can vary. If two atoms both have the same number of protons and neutrons they are the same isotope. If they have the same number of protons and different numbers of neutrons they are different isotopes of the same element. Isotopes are designated by their element, and the total number of neutrons and protons combined.
Some isotopes are stable: as long as nothing happens to them, such as an encounter with a passing solo neutron, they will last for the lifetime of the universe. However, most elements have multiple unstable isotopes that will slowly decay over time, releasing radiation and turning into something quite different.
For example, carbon’s most common is carbon-12, made up of six protons and six neutrons. That’s a suitable balance of the two for stability, and every carbon-12 atom will last forever in most environments. That’s just as well for us, as having one of the most common elements in our body being radioactive would be a problem. Carbon-13, with a seventh neutron, forms more rarely but is also stable.
However, carbon-14 also exists in nature, and we can make carbon-11 in the laboratory. Both are radioactive, meaning they will decay, but carbon-11 does so a great deal more quickly. It’s here the concept of half-life comes in.
The length of time it will take any individual atom to decay can never be predicted, but statistical predictions of large samples can be very precise.
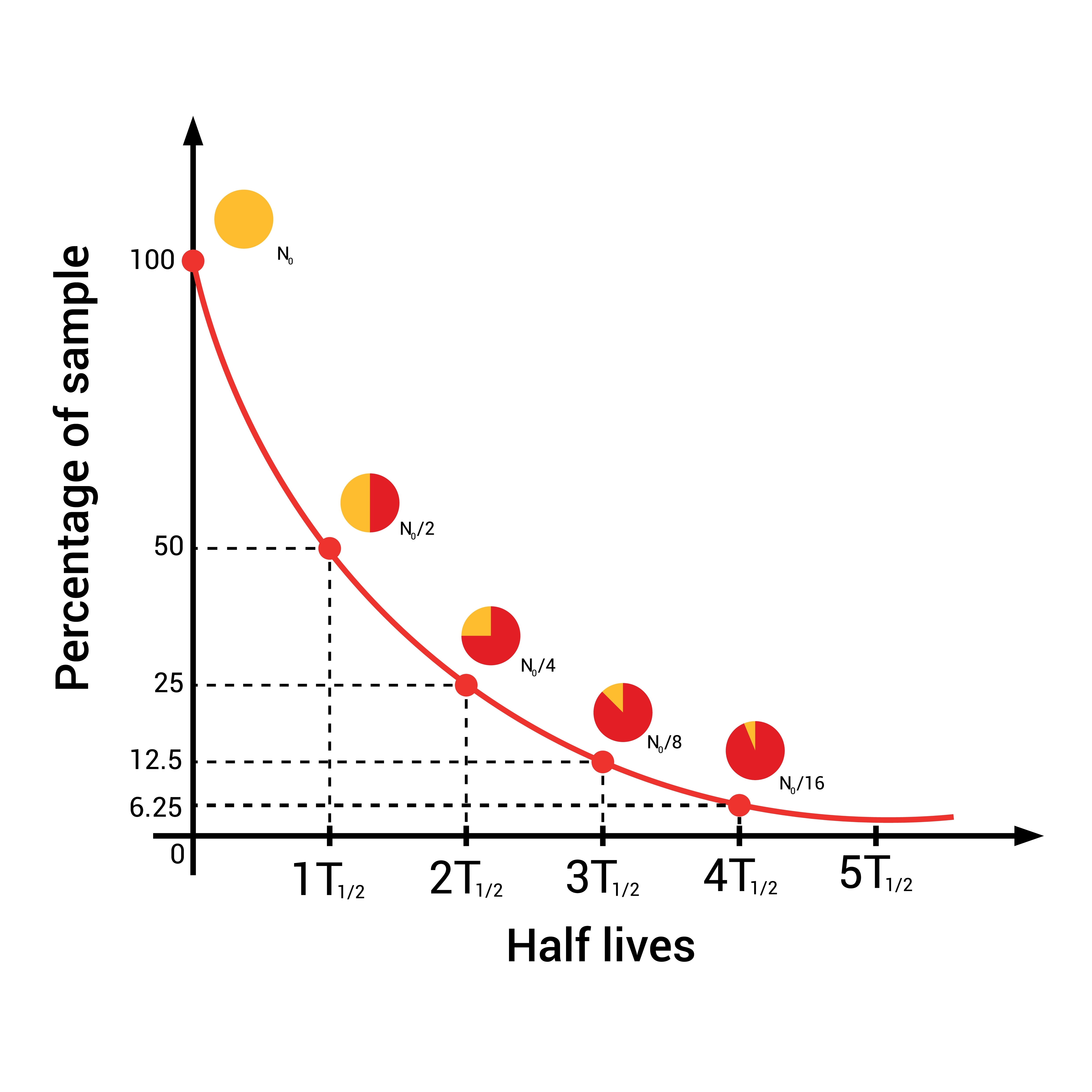
Image Credit: zizou7/Shutterstock.com
For any substantial sample of a radioactive isotope, half the atoms will decay in a period known as that isotope’s half-life. If you suitably store a kilogram of carbon-14, 5,700 years later your heirs will have 500 grams of carbon-14, and 500 grams of nitrogen-14. In between, vast numbers of beta particles (fast-moving electrons) will be released as half the atoms transform from one atom to another.
Half a carbon-11 sample, on the other hand, turns into boron-11 in just 20 minutes, which is why we have to make it ourselves. Any that forms naturally goes very fast.
You might expect that in another 5,700 years, the rest of the carbon-14 would have also decayed, leaving you with nothing but nitrogen, but that’s not how it works. Instead, half the remaining carbon-14 will decay in that time, leaving 250 grams behind. A further 5.7 millennia and there’ll be 125 grams, and so on.
Only when millions of years have passed and you are down to your last few carbon atoms does it start to get unpredictable – with such a small sample size you might lose most of the carbon remaining, or significantly less than half, even though half is the most likely outcome.
Why It Matters
Scientifically, half-lives are crucial for our ability to determine objects’ ages. Plants and photosynthetic algae absorb carbon from the atmosphere, with small amounts of carbon-14 captured in proportion to its presence there. Until recently, this meant the carbon-14 in living plant tissue, and living animals that fed on those plants, was constant. Once the plant or animal dies, however, the carbon-14 starts decaying, while the carbon-12 and -13 stay the same. If you find an object with half as much carbon-14 relative to stable carbon isotopes as was in the historic atmosphere, it means it’s 5,700 years old. A quarter as much means it’s 10,400 years old.
By measuring the amount of carbon-14 we became able to date our ancestors’ fossils for the first time, a crucial step to understanding our evolution. It’s also used to test the authenticity of objects claimed to be ancient relics.
Carbon dating has its limits, however. It can’t be used to measure the ages of objects that are millions of years old, because there will be so little carbon-14 left. On the other hand, in recent years humanity has messed with atmospheric carbon ratios. Previously, most carbon-14 was formed through the products of cosmic rays hitting atmospheric nitrogen atoms. The carbon released by burning fossil fuels has little carbon-14, therefore lowering the atmospheric ratio, while nuclear tests produce enough to raise the global average. These two human activities have worked against each other, but both changed the ratios, which in the future will make it hard to estimate the ages of objects from our times using carbon alone.
Fortunately, however, there are a lot of other radioactive isotopes that can be used for dating, some of which decay much more slowly, allowing us to estimate the age of much older objects.
With a half-life of thousands of years, so few carbon-14 atoms decay in any given year we don’t suffer much from its presence in our organs. The same is not true, however, for some atoms with shorter half-lives. Isotopes such as strontium-90, caesium-137, and cobalt-60 have half-lives short enough that they produce a lot of radiation, but long enough that when an accident causes their release we can’t easily wait for the radiation to go away. When the radiation released in their decays is powerful enough to be dangerous, as it is for these isotopes or the products they decay to, that’s a major problem.
On the other hand, isotopes with short half-lives can be useful as well. We use them for medical imaging, tracking the radiation they release to identify problems in the human body, or to release particles we need for scientific experiments. Often, it’s a matter of searching for an isotope with just the right half-life for the job.
The concept of a half-life has other applications as well. Medications, vitamins, and poisons can have a half-life in the body, with half being flushed out or broken down in a specific period of time. For the first two, this allows us to calculate how quickly we need to replace them.
Source Link: What Are Isotopes’ Half-Lives And Why Do They Matter?