Ever wondered why a sudden reversal of opinion is called a “full 180”? Or why a gaming company might call their new product an “Xbox 360” to imply it can offer the full range of entertainment options?
Like everything great in the world, it all comes down to math – specifically, geometry. Both of these throwaway phrases are in fact references to the number of degrees in a single rotation – or to put it in slightly less math-y terms, the number of degrees in a full circle.
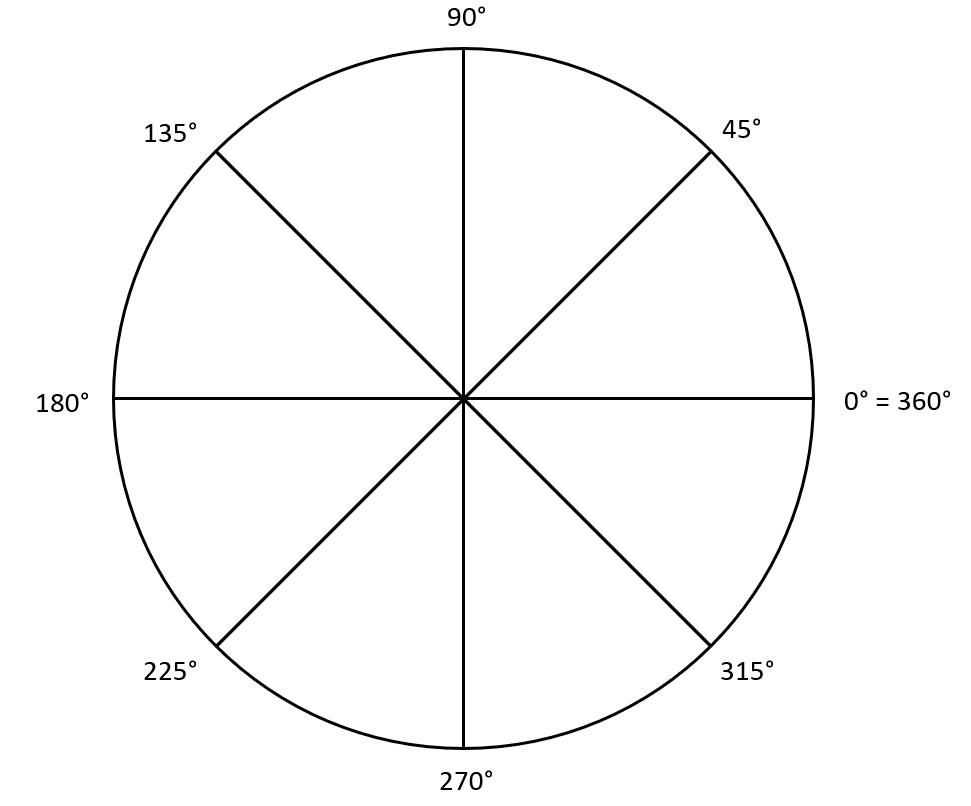
A circle split into eight segments of 45 degrees each.
Image credit: IFLScience
But have you ever wondered why, exactly, we decided to split a circle into such a seemingly arbitrary number? In what other situation (outside of “being American”) would somebody decide to make something like 360 the base value for such an important system?
Why are there 360 degrees in a circle?
For the answer to that, we have to go back to the very beginnings of math itself – back before it was even math at all, really. If you want to know who to blame, look no further than the Ancient Babylonians: they were almost certainly the first to split a circle into 360 equal degrees, and it probably happened around 2400 BCE.
So, case closed, right? There are 360 degrees in a circle because that’s what the Ancient Babylonians decided to do some 4,500 years ago, and we just never decided to update it!
Oh, you wanted more of an explanation than that? Well… now we might have a problem.
The truth is that, like so many decisions made multiple millennia in the past, we don’t know for sure what prompted the choice to set 360 as the number of degrees in a circle. But that doesn’t mean we don’t have a few hypotheses, ranging from mathematical ease to geometric beauty to astronomical serendipity.
The astronomical argument
We all come to math via different routes. For the ancient Greeks, it was through geometry, the study of the earth; in India, the subject came with a distinctly more divine flavor.
For the ancient Babylonians, the true purpose of math lay somewhere in the middle: it was a way to figure out the night sky.
“The heavenly phenomena were of great importance to the Babylonians,” wrote Chris Linton, Professor of Applied Mathematics at Loughborough University, in his 2004 book From Eudoxus to Einstein: A History of Mathematical Astronomy.
“They were perceived as omens and just about every possible astronomical event had some significance,” he explained. “For example, when it came to the retrograde motion of the planets it was not simply the retrograde motion itself, but also where it took place with respect to the stars that was important.”
For example, he noted, should Mars leave the constellation Scorpius, your average Babylonian wouldn’t start talking about how you suddenly have an excuse for being more stubborn than usual or something – it was a cosmic sign that you should put your guard up, because bad things were likely to happen.
In a world before the scientific revolution, this kind of, well, magic was really the only control people could wield over their fate. That meant it was important to get it right: to properly figure out the upcoming omens spelled out in the stars, these ancient astronomers would need precision – and luckily, there was a base unit ready and waiting for them to snap up.
“The Babylonians are… responsible for dividing the circle up into 360 equal parts, which we call ‘degrees’,” Linton wrote. “[This] seems to have been due to the length of the year being about 365 days, and so in one day the Sun moves about 1° with respect to the stars.”
In a year, therefore, the sun would have moved a total of about 360 degrees – and in doing so, had returned to its original position. It had, you might say, come full circle.
The divisibility argument
The Babylonians’ obsession with measuring the skies like this resulted in an astronomical sophistication that far outpaced any other contemporary civilization – and quite a few that came after it, too. All the way up to the 16th century, in fact, Western astronomers were using methods of recording planetary movement that were more or less identical to those used by their 3,000-year-old predecessors.
Why? In fact, this was thanks to yet another quirk of Babylonian math: their counting system. Unlike our own, which is decimal or base 10, theirs was sexagesimal – base 60.

Babylonian numerals one through 59.
It’s not as arbitrary a choice as it sounds. “The reason for the use of 60 is unclear, but it may well have been because 60 is divisible exactly by lots of small integers, so many calculations can be done without the use of fractions,” Linton explained.
That’s a major benefit in a pre-Zeno mathscape. Compare 60 to 10, for example: you can divide the first equally into three and get a whole number, 20, but not the latter; the same goes for dividing by four and six, neither of which have integer solutions for us, but to the Babylonians were extremely simple to work out.
And if 60 is a useful base for working out divisions, then 360 – that is, 60 multiplied by 6 – is even better. To modern mathematicians, it’s what’s known as a superior highly composite number: with no fewer than 24 divisors, it can be split into two, three, four, five, six, eight, nine, 10, 12, 15, 18, 20, 24, 30, 36, 40, 45, 60, 72, 90, 120, 180 and, of course, 360 itself, without ever leaving a remainder.
It’s an incredibly useful property. Try splitting the world into its 24 time zones using 100 degrees in a revolution – you’ll end up needing to measure out 4.166666… recurring degrees of longitude, which is tedious for a cartographer and impossible for a mathematician. With 360 as your starting point, however, it’s a nice round 15 degrees per time zone (or at least, it would be if people stopped messing with them.)
Of course, this line of thought does raise another question: why multiply by six in particular? Again, this is something we can only really speculate about – but it may have something to do with the Greek geometers who inherited the Babylonian mathematical traditions.
See, if there was one thing the earliest Greek geometers loved, it was triangles. If there were two things they loved, it was triangles and symmetry. Now, if you take a circle and draw two radii coming out from the center, then connect them by a third line equal to the length of that same radius – well, you’ll have drawn yourself an equilateral triangle. Three equal sides; three equal angles; every angle 60 degrees.
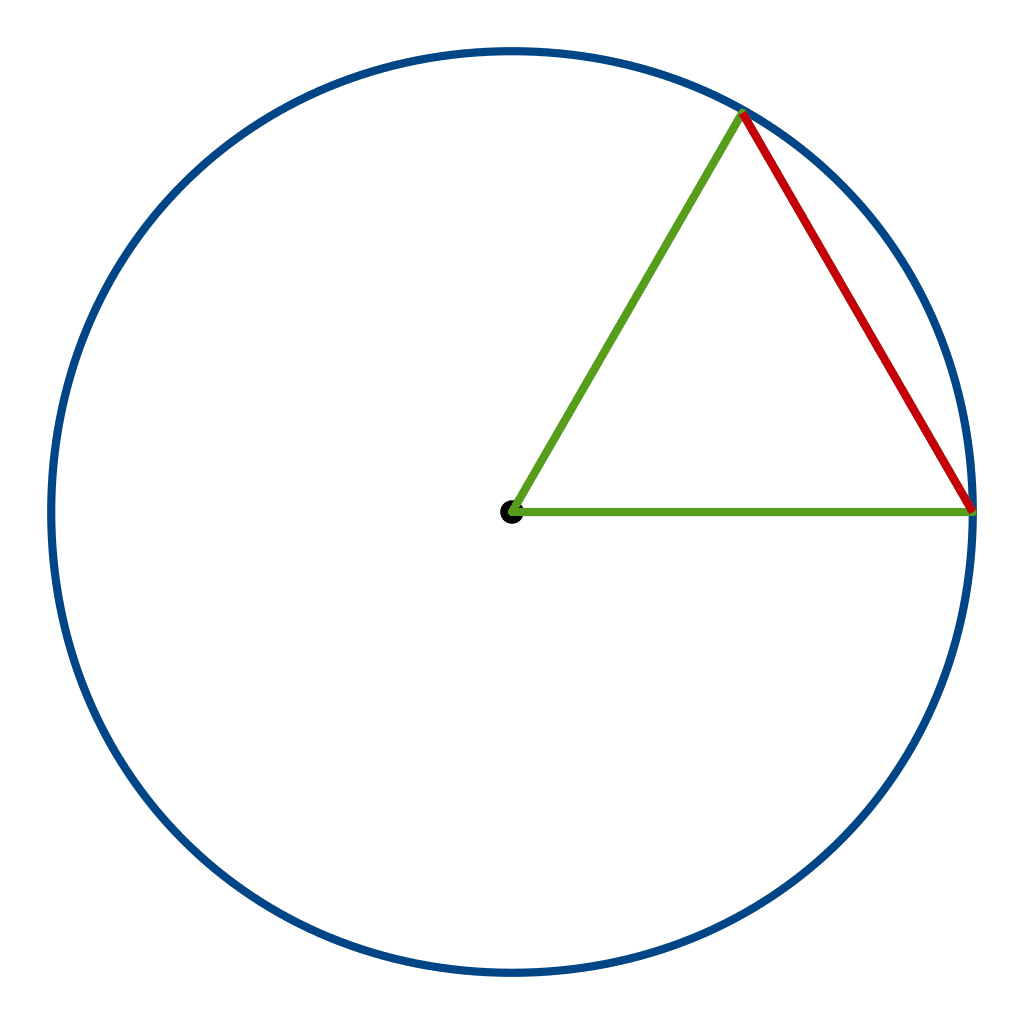
An equilateral triangle inscribed inside a circle.
Fit six of those triangles together, and they will span the entire circle – making this six-times-60 setup a natural foundation for the entire world of geometry.
An even wackier base unit
Of course, in math things can always be made more complicated, and 360 could only last so long as the number of units in a full rotation before it got swapped out for something even more unwieldy.
Enter the radian – another way of measuring angles, used at least since the 1400s by Islamic mathematicians, but only formalized as a concept in the 18th century. For anything past basic geometry, it’s the radian and not the degree that most scientists will reach for – and for good reason: they are a more natural unit from a mathematical perspective, they produce much more elegant and beautiful formulations of results, and they can simplify some calculations that would otherwise be pretty weird and incomprehensible if using degrees.
But from the outside, radians look even worse than degrees. Why? Because the number of radians in a full circle is… 2π.
That’s right, π – as in, the irrational, transcendental number that’s impossible to write using actual digits. So, next time you’re wondering why you have to work with a number as annoying as 360 to deal with your geometry homework, just remember: it could be worse.
After all – the equilateral triangle probably wouldn’t have caught on so well if we all had to remember its internal angles as 1.0471975511965977461542144610931676280657231331250352736583148641 rad, would it?
Source Link: Why Are There 360 Degrees In A Circle, Instead Of Something Useful Like 100?